
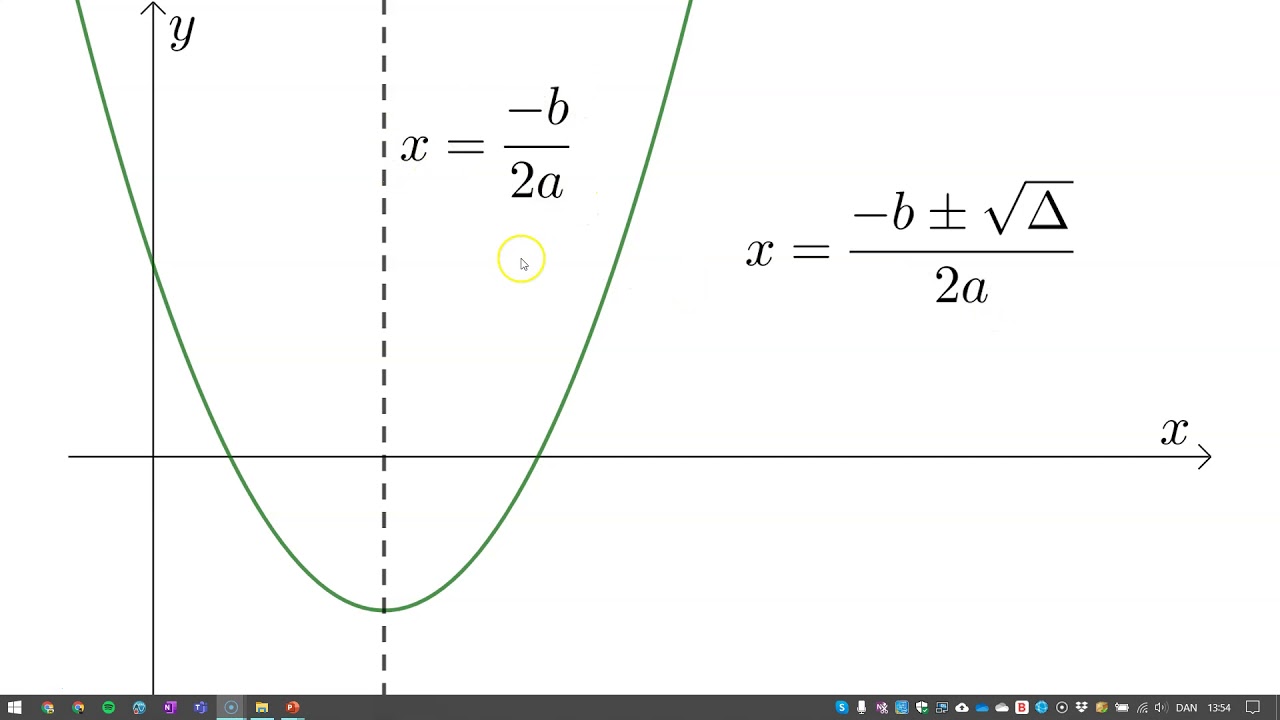
Just enter your example and it will be solved. To find the vertex (h, k) of a parabola that is in standard form y ax2 + bx + c: Use h -b/2a for finding h Substitute x h in the given equation to find k. Example:Īnd how is the general formula for the vertex point? Btw: Whenever there is a negative number in front of the, the parabola is open downward.
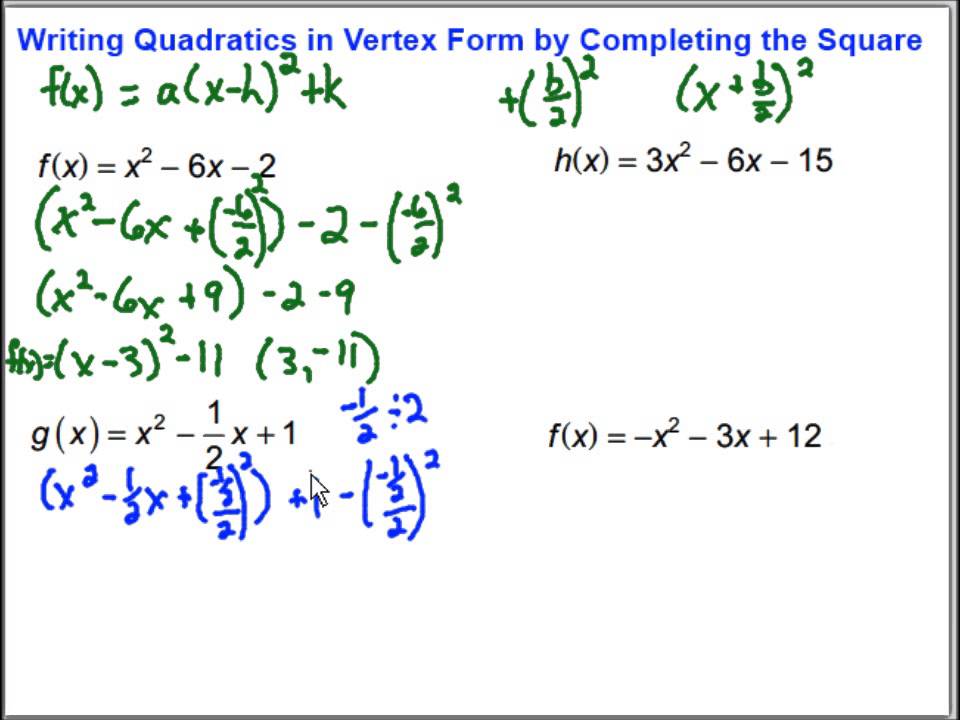
(Unfortunately, many people do not think about such stuff and simply use the binomial formula even if it is not possible… More unfortunately, terms cannot cry ""OUCH!"", but just math teachers can when they see such a calculation.) And if there is a minus in front of the ? is a vertical parabola with axis of symmetry parallel to the y axis and has a vertex V with coordinates (h, k), x - intercepts when they exist and a y - intercept as shown below in the graph. The graph will have one of two shapes, and the a value tells which shape it will be. It is important to factor out first and complete the square afterwards. Review Vertex and Intercepts of a Quadratic Functions The graph of a quadratic function of the form. Date: Finding the Vertex Finding the Vertex Vocabulary and basic facts The graph of any quadratic function f(x) ax2 + bx + c is called a parabola. And if there is a number in front of the ? So simply add the right number and subtract it at the same time. This does only work if there is the right number (the number completing the square). Furthermore, one sees from this calculation that you just have to use the binomial formula backwards: Build a binomial formula out of the function term. :PĪs you can see, the x-coordinate of the vertex equals the number in brackets, but only up to change of signs. Use the vertex form, ya(xh)2+k y a ( x - h ) 2 + k, to determine the values of a a, h h, and k k.
Here is an example:ĭein Browser unterstützt den HTML-Canvas-Tag nicht. You have to complete the square: Take the number in front of x, divide it by and square the result. This means: If the vertex form is, then the vertex is at (h|k). From the vertex form, it is easily visible where the maximum or minimum point (the vertex) of the parabola is: The number in brackets gives (trouble spot: up to the sign!) the x-coordinate of the vertex, the number at the end of the form gives the y-coordinate. The vertex form is a special form of a quadratic function.


 0 kommentar(er)
0 kommentar(er)
